Time Period of Pendulum of Infinite Length

We all know the formula for calculating the time period of an ideal simple pendulum which is T = 2π√(l/g) [for small oscillations] where l is the length of the pendulum and g is the acceleration due to gravity.

Now consider the case when the length of the simple pendulum is very large i.e. tending towards infinity (In earth's perspective, we can say that the length of Pendulum is very very large than the earth's radius). In such a case can we apply the above stated formula to get the time period? The answer is no as the same would not be valid for this case. In fact that formula itself is just a special case of a more general formula. The expression T = 2π√(l/g) is valid only when l <<< R where R represents radius of the earth. We cannot apply it to obtain the time period of a pendulum whose length is comparable to the earth's radius.

Let's proceed to derive a more general expression of time period by taking a general case where length of pendulum is comparable to earth's radius as shown below in the diagram. 

Let's slightly deflect the pendulum and release so that it starts making small oscillations. Now analyze the dynamics of the bob when it is at some general deflection angle Ɵ. At this angular position we get two forces acting on the bob viz. Tension (T) by the thread and gravity mg. One can observe that the line of action of tension T passes through the point of suspension O and therefore torque of T about point O is zero. On the other hand line of action of gravity (mg) crosses through the centre of the earth and hence therefore will produce some restoring torque about point O.

This is the required most general formula for time period of an ideal simple pendulum for small oscillations. We can now take some special cases:

Case 1 : l <<< R (This is the case of a usual simple pendulum whose length is negligible compared to the earth's dimensions) 

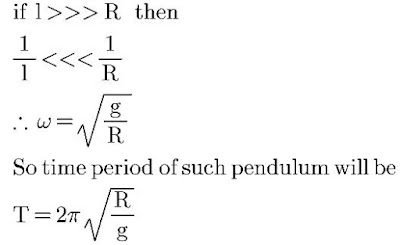
Case 2 : l >>> R (This is the case of a pendulum having large length)

This is our required expression for calculating the time period of a pendulum whose length approaches infinity. On substituting the values of average radius of earth R = 6400 km and acceleration due to gravity g = 9.8 m/s², the time period comes out to be nearly equal to 84.6 minutes.